Understanding Congruent Triangles

In this Lesson you will learn everything pertaining to Congruence and Congruent Triangles:

Understanding the word Congruence:
Yes, it is indeed necessary to first have a clear understanding of the word, Congruence. Once this is done, everything else falls in place. It is just like putting the first button correct in a shirt, and though this is considered a simple task yet, most will prefer to avoid buttoning the shirt from the top, and resort to starting from the last button. Now coming back to the word, Congruence, congruence means to concur, to agree, to correspond. We think, these three synonyms would serve the best in understanding the terminology. Thus, in brevity, we are trying to find some features among triangles that are agreeable. Now with this in mind, let us embark to scale deeper waters.

When are two Triangles said to be Congruent:
Two Triangles are said to be congruent if all of their three corresponding sides are Equal together with all of their three corresponding angles being Equal (Equal is the all important word) in measure. These triangles can be slided, rotated, flipped and turned to look identical. The illustration given below shows two triangles having their Sides and their Angles, all being equal. Hence we can safely conclude that they are congruent to each other.

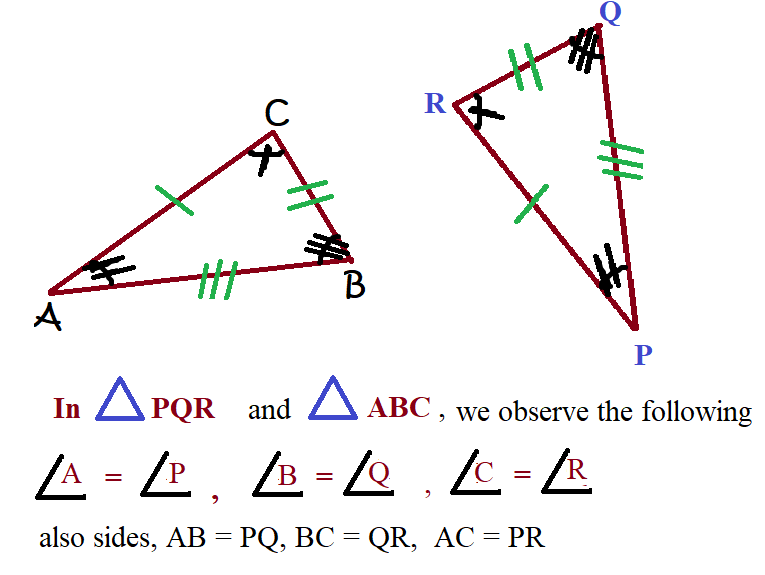
How to represent Congruent Triangles:
In the above Example clearly the given triangles are congruent. But as we all know, Mathematics uses notations and symbols in all representation and hence it becomes imperative to know them. We give below the correct way to represent when two triangles are found to be congruent.

Conditions of Congruence:
Now that we have got the understanding on Congruent triangles, let us now head on to study the concept in detail. It is imperative to understand that several observable characteristics can be employed to determine, whether or not two triangles are congruent. We group these observable characteristics and call them as Criterion. Each criterion will consist of certain predefined characteristics, which ought to be present in the triangles, for congruence to result. Now herein we state these criterion, along with the set of characteristics they behold.

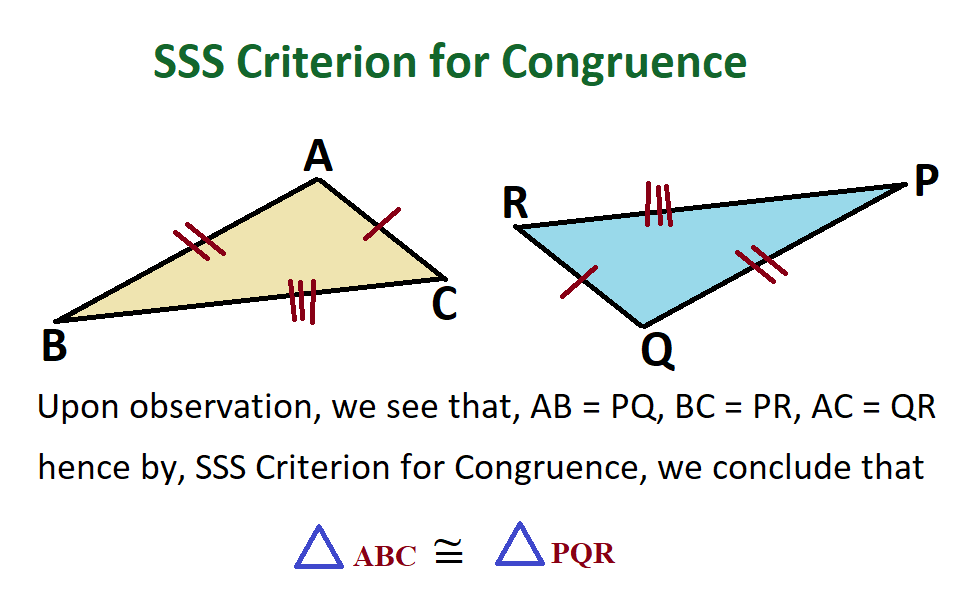
SSS Criterion for Congruence:
This criterion is considered the easiest to spot. The SSS criterion stands for Side-Side-Side criterion. Under this criterion, the two triangles under question will be congruent if and only if the three sides of the first triangle matches exactly in length with the three sides of the second triangle.

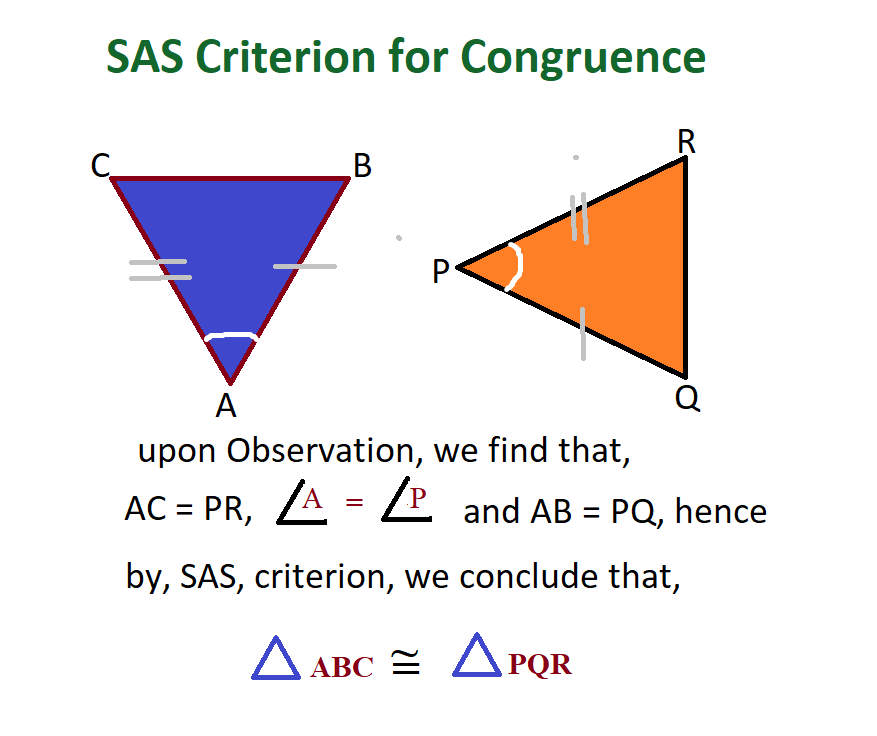
SAS Criterion for Congruence:
If any two sides and the angle included between the sides of the first triangle should be equal to the second triangle's two sides and the angle that lies between these two sides. If thise occurs, then then the two triangles are said to be congruent by SAS criterion.

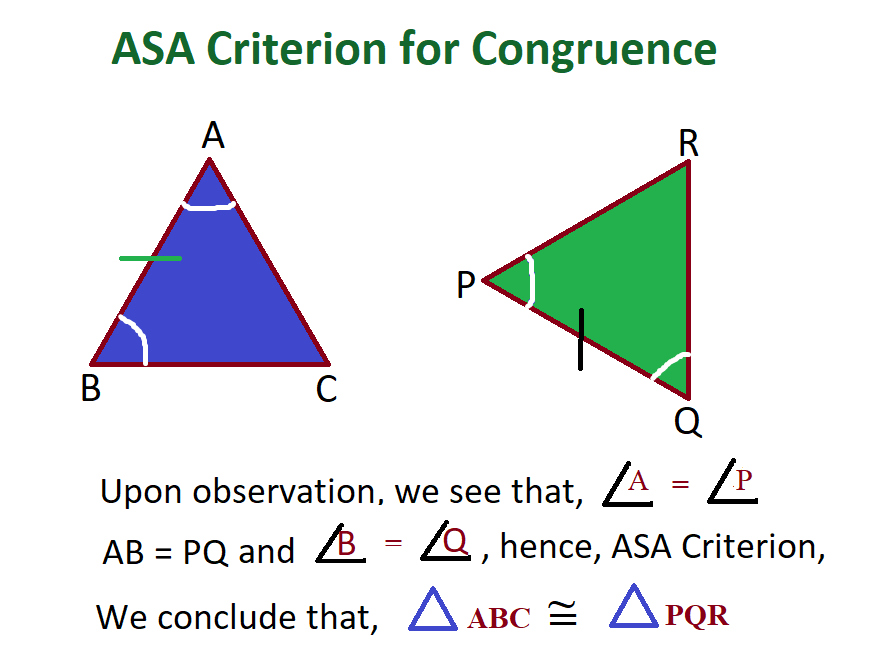
ASA Criterion for Congruence:
ASA criterion stands for Angle-Side-Angle criterion. Under the ASA criterion, two triangles are congruent only if any two angles and the side included between them of first triangle equals the two angles and the side included in between, belonging to the second triangle.

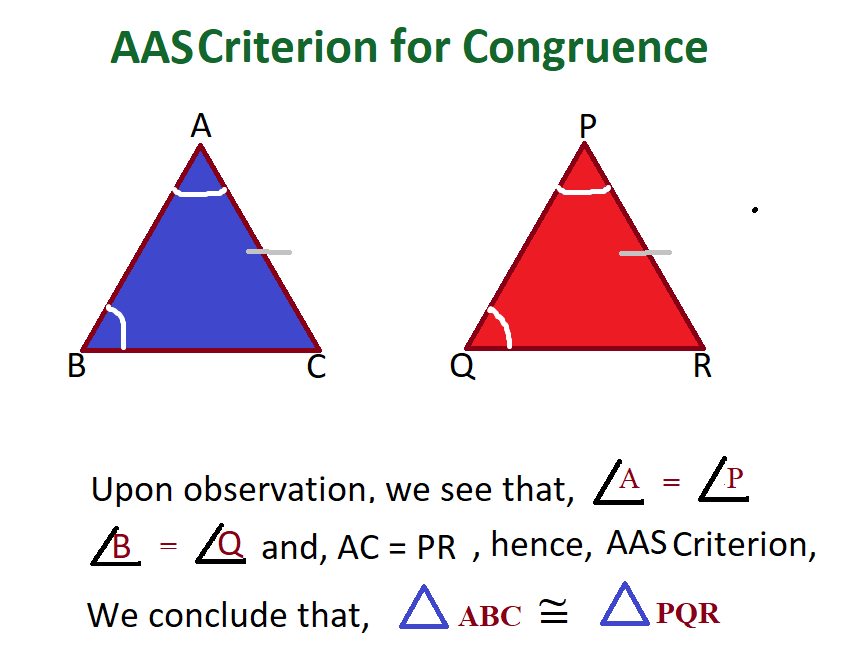
AAS Criterion for Congruence:
AAS criterion stands for Angle-Angle-Side criterion. Under the AAS criterion, two triangles are congruent if any two angles and the non-included side of the first triangle equals the corresponding angles and the non-included side of the second triangle.

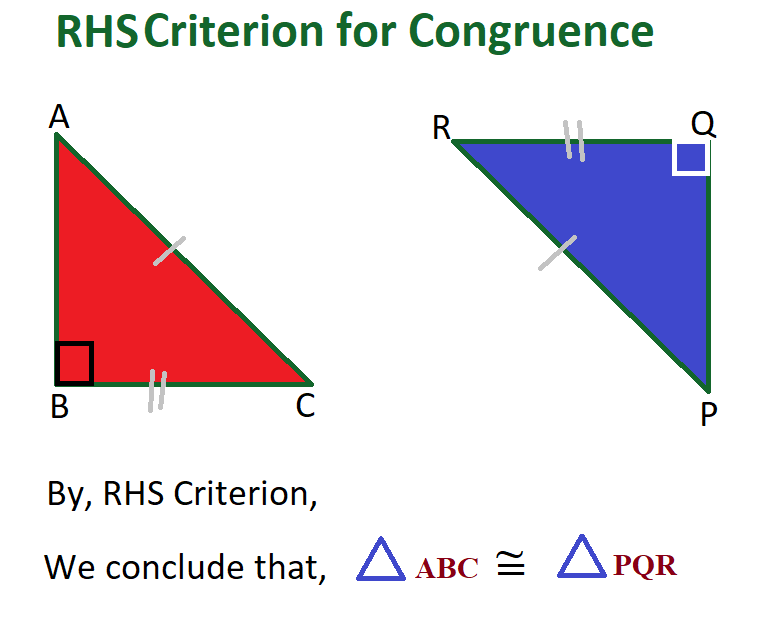
RHS Criterion for Congruence:
RHS criterion stands for Right angle-Hypotenuse-Side congruence criterion. Under this criterion, two triangles are congruent,If the Hypotenuse and a Side of the first Right- angled triangle is equivalent to the Hypotenuse and a Side of the second Right- angled triangle.